how to find area of trapezoid

Area of Trapezium

Here we will learn how to use the formula to find the area of trapezium.

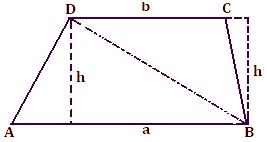
Area of trapezium ABCD = Area of ∆ ABD + Area of ∆ CBD

= 1/2 × a × h + 1/2 × b × h

= 1/2 × h × (a + b)

= 1/2 (sum of parallel sides) × (perpendicular distance between them)

$Area of trapezium$

Worked-out examples on area of trapezium

1. The length of the parallel sides of a trapezium are in the rat: 3 : 2 and the distance between them is 10 cm. If the area of trapezium is 325 cm², find the length of the parallel sides.

Solution:

Let the common ration be x,

Then the two parallel sides are 3x, 2x

Distance between them = 10 cm

Area of trapezium = 325 cm²

Area of trapezium = 1/2 (p₁ + p₂) h

325 = 1/2 (3x + 2x) 10

⇒ 325 = 5x × 5

⇒ 325 = 25x

⇒ x = 325/25

Therefore, 3x = 3 × 13 = 39 and 2x = 2 × 13 = 26

Therefore, the length of parallel sides area are 26 cm and 39 cm.

2. ABCD is a trapezium in which AB ∥ CD, AD ⊥ DC, AB = 20 cm, BC = 13 cm and DC = 25 cm. Find the area of the trapezium.

$find the area of trapezium$

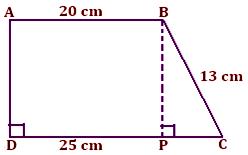
Solution:

From B draw BP perpendicular DC

Therefore, AB = DP = 20 cm

So, PC = DC - DP

= (25 - 20) cm

= 5 cm

Now, area of trapezium ABCD = Area of rectangle ABPD + Area of △ BPC

△BPC is right angled at ∠BPC

Therefore, using Pythagoras theorem,

BC² = BP² + PC²

13² = BP² + 5²

⇒ 169 = BP² + 25

⇒ 169 - 25 = BP²

⇒ 144 = BP²

⇒ BP = 12

Now, area of trapezium ABCD = Area of rectangle ABPD + Area of ∆BPC

= AB × BP + 1/2 × PC × BP

= 20 × 12 + 1/2 × 5 × 12

= 240 + 30

= 270 cm²

3. Find the area of a trapezium whose parallel sides are AB = 12 cm, CD = 36 cm and the non-parallel sides are BC = 15 cm and AG = 15 cm.

$examples on area of trapezium$

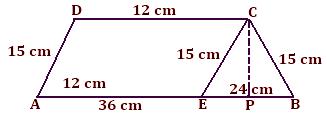
Solution:

In trapezium ABCD,draw CE ∥ DA.

Now CE = 15 cm

Since, DC = 12 cm so, AE = 12 cm

Also, EB = AB - AE = 36 - 12 = 24 cm

Now, in ∆ EBC

S = (15 + 15 + 24)/2

= 54/2

= 27

= √(27 × 12 × 12 × 3)

= √(3 × 3 × 3 × 3 × 2 × 2 × 2 × 2 × 3 × 3)

= 3 × 3 × 3 × 2 × 2

= 108 cm²

Draw CP ⊥ EB.

Area of ∆EBC = 1/2 × EB × CP

108 = 1/2 × 24 × CP

108/12 = CP

⇒ CP = 9 cm Therefore, h = 9 cm

Now, area of triangle = √(s(s - a) (s - b) (s - c))

= √(27 (27 - 15) (27 - 15 ) (27 - 24))

Now, area of trapezium = 1/2(p₁ + p₂) × h

= 1/2 × 48 × 9

= 216 cm²

4. The area of a trapezium is 165 cm² and its height is 10 cm. If one of the parallel sides is double of the other, find the two parallel sides.

Solution:

Let one side of trapezium is x, then other side parallel to it = 2x

Area of trapezium = 165 cm²

Height of trapezium = 10 cm

Now, area of trapezium = 1/2 (p₁ + p₂) × h

⇒ 165 = 1/2(x₁ + 2x) × 10

⇒ 165 = 3x × 5

⇒ 165 = 15x

⇒ x = 165/15

⇒ x = 11

Therefore, 2x = 2 × 11 = 22

Therefore, the two parallel sides are of length 11 cm and 22 cm.

These are the above examples explained step by step to calculate the area of trapezium.

● Mensuration

Area and Perimeter

Perimeter and Area of Rectangle

Perimeter and Area of Square

Area of the Path

Area and Perimeter of the Triangle

Area and Perimeter of the Parallelogram

Area and Perimeter of Rhombus

Area of Trapezium

Circumference and Area of Circle

Units of Area Conversion

Practice Test on Area and Perimeter of Rectangle

Practice Test on Area and Perimeter of Square

●Mensuration - Worksheets

Worksheet on Area and Perimeter of Rectangles

Worksheet on Area and Perimeter of Squares

Worksheet on Area of the Path

Worksheet on Circumference and Area of Circle

Worksheet on Area and Perimeter of Triangle

Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.